Let $C$ be a smooth plane curve given by the parametric equations $$x=x(t),\quad y=y(t),\quad a\leq t\leq b$$ or equivalent, by the vector equation $\mathbf r (t)=x(t)\,\mathbf i +y(t)\,\mathbf j$. If $f$ is any function of two variables whose domain includes the curve $C$, then the line integral of $f$ along $C$ is \[ \int_Cf(x,y)\,ds=\int_a^bf(\mathbf r (t))\,|\mathbf r' (t)|\,dt. \]
This integral can also be constructed from a Riemann sum. If we divide the parameter interval $[a,b]$ into $n$ subintervals $[t_{i-1},t_i]$ of equal width and we let $x_i=x(t_i)$ and $y_i=y(t_i)$, then the corresponding points $P_i(x_i,y_i)$ divide $C$ into $n$ subarcs with lengths $\Delta s_1,\Delta s_2,\ldots , \Delta s_n$. We choose any point $P^{*}(x_i^{*},y_i^{*})$ in the $i$th subarc. Now we evaluate $f$ at the point $(x_i^{*},y_i^{*})$, multiply by the length $\Delta s_i$ of the subarc, and form the sum $$\sum _{i=1}^{n}f(x_{i}^{*},y_{i}^{*})\,\Delta s_i$$ which is similar to a Riemann sum. Thus the line integral of $f$ along $C$ can also be defined as \[ \int_Cf(x,y)\,ds=\lim_{n\rightarrow \infty}\sum _{i=1}^{n}f(x_{i}^{*},y_{i}^{*})\,\Delta s_i \]
If $f(x,y)\geq 0$, then $\int_Cf(x,y)\,ds$ represents the area of one side "fence" or "curtain", whose height above the point $(x,y)$ is $f(x,y)$.
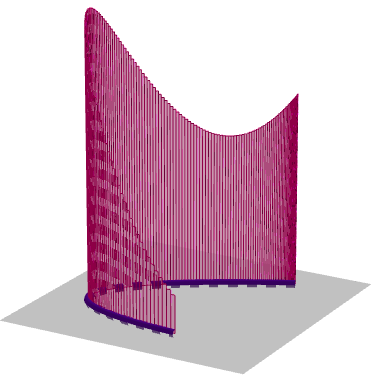
The following simulation shows approximate values for the line integral of a scalar function using a middle Riemann sum. This means that we choose $P^{*}(x_i^{*},y_i^{*})$ as the middle point in the $i$th subarc to form the sum of rectangles with
Things to try:
The following file contains activities and problems associated with the simulation.
The University of Queensland